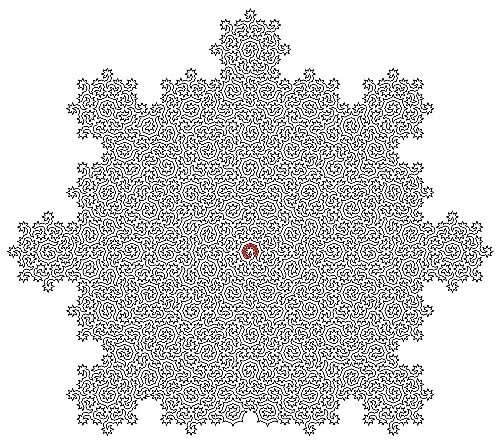
The image shows a Hamiltonian cycle (visited atoms are not shown). Credit: University of Bristol
In new research, physicists have harnessed the power of chess to design a group of complex mazes that could ultimately be used to address some of the world’s most pressing challenges.
Their unique labyrinthine creations, inspired by the movements of a knight on a chessboard, could help solve other notoriously difficult problems, including streamlining industrial processes from carbon capture to fertilizer production. The study has been accepted for publication by Physical examination X and is displayed on the arXiv preprint server.
Lead author Dr Felix Flicker, a lecturer in physics at the University of Bristol, said: “When we looked at the shapes of the lines we constructed, we noticed that they formed incredibly complex mazes. The size of subsequent mazes increases exponentially, and there are an infinite number of them.”
On a knight’s turn, the chess piece (which jumps two squares forward and one square to the right) visits each square on the board once before returning to its starting square. This is an example of a “Hamiltonian cycle”: a loop through a board visiting all stopping points once.
Theoretical physicists, led by the University of Bristol, have constructed an infinite number of ever-larger Hamiltonian cycles in irregular structures that describe an exotic material known as quasicrystals.
The atoms in quasicrystals are arranged differently than those in crystals such as salt or quartz. While the atoms in crystals repeat at regular intervals, like the squares on a chessboard, the atoms in quasicrystals do not.
Instead, they do something a little more mysterious: Quasicrystals can be described mathematically as slices of crystals that live in six dimensions, as opposed to the three of our familiar universe.
Only three natural quasicrystals have been discovered, all in the same Siberian meteorite. The first artificial quasicrystal was accidentally created during the 1945 Trinity test, the atomic bomb explosion dramatized in the Oppenheimer film.
The group’s Hamiltonian cycles visit every atom on the surface of certain quasicrystals exactly once. The resulting paths form particularly complex labyrinths, described by mathematical objects called “fractals.”
These trajectories have the special property that an atomically sharp pencil could trace straight lines connecting all neighboring atoms, without the pencil lifting or the line crossing itself. This finds applications in a process known as scanning tunneling microscopy, where the pencil is an atomically sharp microscope tip capable of imaging individual atoms.
Hamiltonian cycles are the fastest routes the microscope can follow. This is useful because it can take a month to obtain a state-of-the-art scanning tunneling microscopy image.
The problem of finding Hamiltonian cycles in general settings is so difficult that its solution would automatically solve many important problems that remain to be solved in the mathematical sciences.
Dr Flicker added: “We show that some quasicrystals are a special case in which the problem is surprisingly simple. In this context, we therefore make some seemingly impossible problems solvable. This could include practical goals spanning different scientific domains.”
For example, adsorption is a key industrial process in which molecules adhere to the surface of crystals. So far, only crystals are used for industrial adsorption. If the atoms on a surface admit a Hamiltonian cycle, flexible molecules of the right size can stack with perfect efficiency by placing themselves along these atomic labyrinths.
The research results demonstrate that quasicrystals can be very efficient adsorbers. One use of adsorption is carbon capture and storage, in which CO2 molecules are prevented from entering the atmosphere.
Shobhna Singh, co-author and PhD researcher in physics at Cardiff University, said: “Our work also shows that quasicrystals may be better than crystals for some adsorption applications. For example, flexible molecules will find more ways to land on the irregularly arranged atoms of quasicrystals. Quasicrystals are also brittle, meaning they break easily into tiny grains. This maximises their adsorption surface area.”
Efficient adsorption could also make quasicrystals surprising candidates for catalysis, which increases industrial efficiency by reducing the energy of chemical reactions. For example, adsorption is a key step in the Haber catalysis process, used to produce ammonia fertilizer for agriculture.
More information:
Shobhna Singh et al., Hamiltonian cycles on Ammann-Beenker tilings, arXiv (2023). DOI: 10.48550/arxiv.2302.01940
Provided by the University of Bristol
Quote:Scientists Create World’s Most Incredibly Difficult Maze With Future Potential to Boost Carbon Capture (2024, July 2) Retrieved July 3, 2024, from https://phys.org/news/2024-07-scientists-world-amazingly-difficult-maze.html
This document is subject to copyright. Apart from any fair dealing for the purpose of private study or research, no part may be reproduced without written permission. The content is provided for informational purposes only.